Fields

Although there are many different geophysical methods, small-scale surveys
all tend to be rather alike and involve similar, and sometimes ambiguous,
jargon. For example, the word base has three different common meanings,
and stacked and field have two each.
Measurements in geophysical surveys are made in the field but, unfortunately,
many are also of fields. Field theory is fundamental to gravity,
magnetic and electromagnetic work, and even particle fluxes and seismic
wavefronts can be described in terms of radiation fields. Sometimes ambiguity
is unimportant, and sometimes both meanings are appropriate (and
intended), but there are occasions when it is necessary to make clear distinctions.
In particular, the term field reading is almost always used to identify
readings made in the field, i.e. not at a base station.
The fields used in geophysical surveys may be natural ones (e.g. the
Earth’s magnetic or gravity fields) but may be created artificially, as when
alternating currents are used to generate electromagnetic fields. This leads to
the broad classification of geophysical methods into passive and active types,
respectively.
Physical fields can be illustrated by lines of force that show the field
direction at any point. Intensity can also be indicated, by using more closely
spaced lines for strong fields, but it is difficult to do this quantitatively where
three-dimensional situations are being illustrated on two-dimensional media.
 Vector addition
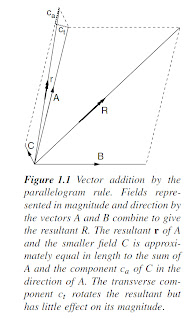
Vector addition (Figure 1.1) must be used when combining fields from different
sources. In passive methods, knowledge of the principles of vector
addition is needed to understand the ways in which measurements of local
anomalies are affected by regional backgrounds. In active methods, a local
anomaly (secondary field) is often superimposed on a primary field produced
by a transmitter. In either case, if the local field is much the weaker of the two
(in practice, less than one-tenth the strength of the primary or background
field), then the measurement will, to a first approximation, be made in the
direction of the stronger field and only the component in this direction of
the secondary field (ca in Figure 1.1) will be measured. In most surveys the
slight difference in direction between the resultant and the background or
primary field can be ignored.

If the two fields are similar in
strength, there will be no simple
relationship between the magnitude
of the anomalous field and the
magnitude of the observed anomaly.
However, variations in any given
component of the secondary field
can be estimated by taking all
measurements in an appropriate
direction and assuming that the
component of the background or
primary field in this direction is
constant over the survey area.
Measurements of vertical rather than
total fields are sometimes preferred
in magnetic and electromagnetic
surveys for this reason.
The fields due to multiple sources
are not necessarily equal to the
vector sums of the fields that would
have existed had those sources
been present in isolation. A strong
magnetic field from one body can
affect the magnetization in another,
or even in itself (demagnetization
effect), and the interactions between fields and currents in electrical and
electromagnetic surveys can be very complex.
 The inverse-square law
Inverse-square law attenuation of signal strength occurs in most branches of
applied geophysics. It is at its simplest in gravity work, where the field due
to a point mass is inversely proportional to the square of the distance from
the mass, and the constant of proportionality (the gravitational constant G)
is invariant. Magnetic fields also obey an inverse-square law. The fact that
their strength is, in principle, modified by the permeability of the medium
is irrelevant in most geophysical work, where measurements are made in
either air or water. Magnetic sources are, however, essentially bipolar, and
the modifications to the simple inverse-square law due to this fact are much
more important (Section 1.1.5).
Electric current flowing from an isolated point electrode embedded in
a continuous homogeneous ground provides a physical illustration of the

significance of the inverse-square law. All of the current leaving the electrode
must cross any closed surface that surrounds it. If this surface is a sphere
concentric with the electrode, the same fraction of the total current will cross
each unit area on the surface of the sphere. The current per unit area will
therefore be inversely proportional to the total surface area, which is in turn
proportional to the square of the radius. Current flow in the real Earth is, of
course, drastically modified by conductivity variations.
1.1.3 Two-dimensional sources
Rates of decrease in field strengths depend on source shapes as well as on
the inverse-square law. Infinitely long sources of constant cross-section are
termed two-dimensional (2D) and are often used in computer modelling to
approximate bodies of large strike extent. If the source ‘point’ in Figure 1.2
represents an infinite line source seen end on, the area of the enclosing (cylindrical)
surface is proportional to the radius. The argument applied in the
previous section to a point source implies that in this case the field strength
is inversely proportional to distance and not to its square. In 2D situations,
lines of force drawn on pieces of paper illustrate field magnitude (by their
separation) as well as direction.

The lines of force or radiation intensity from a source consisting of a homogeneous layer of
constant thickness diverge only near its edges (Figure 1.3). The Bouguer plate of gravity reductions (Section 2.5.1) and the radioactive source with 2π geometry (Section 4.3.3) are examples of infinitely extended layer sources, for which field strengths are independent
of distance. This condition is approximately achieved if a detector is only a short distance
above an extended source and a long way from its edges.

A dipole consists of equal-strength positive and negative point sources a very small distance apart. Field strength decreases as the inverse cube of distance and both strength and direction change with ‘latitude’ (Figure 1.4). The intensity of the field at a point on a dipole
axis is double the intensity at a point the same distance away on the dipole ‘equator’, and in the opposite direction.

Electrodes are used in some
electrical surveys in approximately
dipolar pairs and magnetization is
fundamentally dipolar. Electric currents
circulating in small loops are
dipolar sources of magnetic field.





Exponential decay
Radioactive particle fluxes and seismic and electromagnetic waves are subject
to absorption as well as geometrical attenuation, and the energy crossing

closed surfaces is then less than the energy emitted by the sources they
enclose. In homogeneous media, the percentage loss of signal is determined
by the path length and the attenuation constant. The absolute loss is proportional
also to the signal strength. A similar exponential law (Figure 1.5),
governed by a decay constant, determines the rate of loss of mass by a
radioactive substance.
Attenuation rates are alternatively characterized by skin depths, which
are the reciprocals of attenuation constants. For each skin depth travelled, the
signal strength decreases to 1/e of its original value, where e (= 2.718) is the
base of natural logarithms. Radioactivity decay rates are normally described in
terms of the half-lives, equal to loge2 (= 0.693) divided by the decay constant.
During each half-life period, one half of the material present at its start is lost.

The Second Law and Molecular Behavior

At the present time we are familiar enough with molecules to formulate the Second
Law entirely in relation to an intuitive perception of their behavior. It is easy to see that the
Second Law, as expressed in terms of heat flow in section 11, could be violated with some
cooperation from molecules.
Consider two systems each consisting of a fixed quantity of gas. At the boundary of
each system are rigid, impenetrable, and well insulated walls except for a metal plate made
of a good thermal conductor and located between the systems as shown in Figure 1. The gas
in one system is at a low temperature T1 and in the other at a higher temperature T2. In
terms of molecular behavior the

temperature difference is produced by different distributions of molecules among the
velocities in the molecular states of each system. The number of different velocities,
however, is so large that the high temperature system contains some molecules with lower

speeds than some molecules in the low temperature system and vice versa.
Now suppose we prepare some instructions for the molecules in each system as
shown on the signs in Figure 1. When following these instructions, only the high speed
molecules in the low temperature gas collide with molecules on the surface of the plate, give
up energy to them, and thus create on this surface a higher temperature than in the gas.
The boundary between the gas and the plate then has a temperature difference across it and,
according to our definition, the energy thus transported is heat. In the plate this becomes
thermal energy which is conducted through it because the molecules on its other surface are
at a lower temperature as a consequence of their energy exchanges only with the low speed
molecules in the adjacent high temperature gas system. This constitutes likewise a heat flow
into this system. The overall result is then the continuous unaided transfer of heat from a
low temperature region to one at a higher temperature, clearly the wrong direction and a
Second Law violation. The Second Law therefore, is related to the fact that in completely
isolated systems molecules will never of their own accord obey any sort of instructions such
as these.
                          Microstates in Isolated Systems

To explain why molecules always behave as though instructions of this type are
completely ignored, imagine that we have a fantastic camera capable of making a
multidimensional picture which could show at any instant where all the ultimate particles
in the system are located and reveal every type of motion taking place, indicating its
location, speed, and direction. Every type of distinguishably different action at any moment,
the vibration, twisting, or stretching within molecules as well as their translational and
rotational movements, would be identified in this manner for every molecule in the system.
This picture would thus be a photograph of what we have defined as an instantaneous
microstate of the system.
Now, instead of the instructions on the signs in Figure I, suppose we ask the
molecules to do everything they can do by themselves in a rigid walled and isolated
container where no external arranging or directing operations are possible. We will say,
"Molecules, please begin now and arrange yourselves in a sequence of poses for pictures
which will show every possible microstate which can exist in your system under the
restrictions imposed by your own nature and the conditions of isolation in the container".
If we expressed these restrictions as a list of rules to be followed in assigning molecules to various positions and motions, the list would appear as follows:
1. In distributing yourselves among the various positions and motions for each microstate
picture, do not violate any energy conservation laws. Consequently, because you are in
an isolated system the sum of all your individual translational, vibrational, and
rotational kinetic energies plus all your intermolecular potential energies must always
be the same and equal to the fixed total internal energy of the system.
2. Likewise, do not violate any mass conservation laws. There are to be no chemical
reactions among you so that the total number of individual molecules assigned must
always remain the same.
3. All of you must, of course, remain at all times within the container so the total volume
in which you distribute yourselves must be constant.
4. Do not violate any laws of physics applicable to your particular molecular species. You
must remember that no two of you can have all of your microstate position and motion
characteristics exactly the same otherwise you would have to occupy the same space at
the same time. Furthermore, do not be concerned that there might not be enough
different microstates available for each of you to have a different one. Although you are
numerous, the number of different possible position and motion values is even more
numerous, so that there will never be enough of you to fill all of them and many
possible values will be left unoccupied by a molecule in each picture for which you pose.

The Total Energy Transfer

Because thermodynamic systems are conventionally defined so that no bulk
quantities of matter are transported across their boundaries by stream flow, no energy
crosses the system boundary in the form of internal energy carried by a flowing fluid. With
the system defined in this way the only energy to cross its boundary because of the flow
process is that of work measured by the product of the pressure external to a fixed mass
system in a stream conduit and the volume change it induces in this system. In the case of
diffusion mass transport, as discussed in section 15, the system does not have a fixed mass
but the entire change associated with the diffusion mass transport is given by work
evaluated by computing the product of an external chemical potential and a specific
transported mass change within the system. As a result the combination of heat, work, and
any energy transport by non-thermodynamic carriers includes all the energy in transition
between a system and its surroundings. Energy by non-thermodynamic carriers is that
transported by radiant heat transfer, X-rays, gamma radiation, nuclear particles, cosmic
rays, sonic vibration, etc. Energy of this type is not usually considered as either heat or
work and must be evaluated separately in system where it is involved. Energy transport by
nuclear particles into a system ultimately appears as an increase in thermal energy within
the system and is important in thermodynamic applications to nuclear engineering.
In every application of thermodynamics, however, it is essential that we account for
all the energy in transition across the system boundary and it is only when this is done that
the laws of thermodynamics can relate this transported energy to changes in properties
within the system. In the processes we will discuss, heat and work together include all the
transported energy.